The expected future service is calculated in all accounting Valuations and Core Projections. It is also calculated in funding Valuations and Core Projections in Public, Universal, Canadian, and UK modes. In Valuations & Core Projections, when calculating the average expected future service, the denominator is always based on the head count expected to receive benefits.
The expected future service of active members can be used in Valuation Sets, Deterministic Forecasts, and Stochastic Forecasts to amortize accounting gains & losses, and prior service costs arising from plan amendments. In your Asset & Funding Policy (under the Accounting Methodology topic, Future Amortizations button) you may choose level payments over average future service or declining payments over total future service. You may also choose whether the denominator used to calculate the average will be based on the head count expected to receive benefits or the average head count during the year. (Prior to ProVal version 3.01, the denominator was always calculated as average head count during the year.)
BEGINNING-OF-YEAR DECREMENTS:
For a beginning of year decrement timing assumption, under the average future service option, each employee’s contribution to the average’s numerator and denominator is:
Numerator:
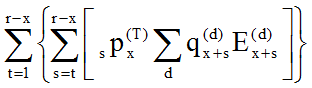
Denominator:
If “Headcount expected to receive benefits” is selected:
If “Average headcount during the year” is selected:
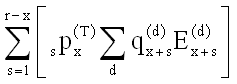
Where:
x = current age on the valuation date;
r = the age when retirement rates first become 100%;
d represents the decrement (i.e. retirement, termination, death or disability);
p(T) = the probability of surviving all decrements;
q(d) = the probability of leaving active service because of decrement d and
E(d) = 1 if a benefit is projected to be payable for decrement d, else 0. (For calculating expected service lives under GASB 68 or 75, E is set to 1 until the last decrement age at which a non-zero benefit is assumed to be payable and zero for all decrement ages thereafter.)
Note that the portion of an employee expected to terminate without benefits is excluded. If no portion of an employee is excluded, because benefits are payable for all decrements at all ages (i.e. E=1 whenever q>0), then the equations simplify to:
Numerator:
Denominator:
If “Headcount expected to receive benefits” is selected: 1.
If “Average headcount during the year” is selected:
Under the total future service option, each employee’s contribution to the total in the year ending at time t is:
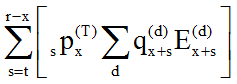
MIDDLE-OF-YEAR DECREMENTS:
For a middle of year decrement timing assumption, the equations include a half-year of service in the year of decrement.
Under the average future service option, each employee’s contribution to the average’s numerator and denominator is:
Numerator:
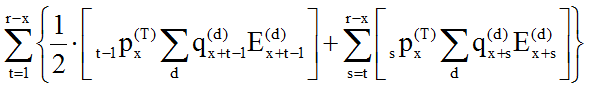
Denominator:
If “Headcount expected to receive benefits” is selected:
, in which the notation is as indicated above (for beginning of year decrements).
If “Average headcount during the year” is selected:
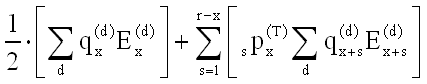, in which the notation is as indicated above (for beginning of year decrements).
Note that the portion of an employee expected to terminate without benefits is excluded. If no portion of an employee is excluded, because benefits are payable for all decrements at all ages (i.e. E=1 whenever q>0), then the equations simplify to:
Numerator:
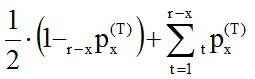
Denominator:
If “Headcount expected to receive benefits” is selected: 1.
If “Average headcount during the year” is selected:
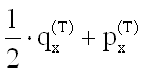
Under the total future service option, each employee’s contribution to the total in the year ending at time t is:
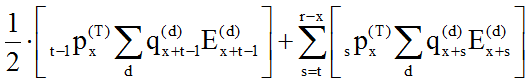
EXAMPLE, with average calculated under denominator option “Headcount expected to receive benefits”:
| Number of actives: | 1 |
| Current age: | 55 |
| Decrements: | mortality q(m) and retirement q(r) |
| Decrements occur at: | beginning of year |
| 100% retirement age: | 62 |
| (A) | (B) | (C) | |||||
| Age | q(m) | E(m) | q(r) | E(r) | q(m)*E(m) + q(r)*E(r) | p(T) | Sum to 62 (A)*(B) |
| 55 | 0.008 | 1 | 0 | 0 | |||
| 56 | 0.009 | 1 | 0 | 0 | 0.009 | 0.992000 | 0.992000 |
| 57 | 0.01 | 1 | 0 | 0 | 0.01 | 0.983072 | 0.983072 |
| 58 | 0.011 | 1 | 0 | 0 | 0.011 | 0.973241 | 0.973241 |
| 59 | 0.012 | 1 | 0 | 0 | 0.012 | 0.962536 | 0.962536 |
| 60 | 0.013 | 1 | 0.2 | 1 | 0.213 | 0.950985 | 0.950985 |
| 61 | 0.014 | 1 | 0.1 | 1 | 0.114 | 0.748425 | 0.748425 |
| 62 | 0 | 0 | 1 | 1 | 1 | 0.663105 | 0.663105 |
| Total | 6.273364 | ||||||
| Average | 6.273364 |
Note that in this example, columns (B) and (C) are the same. This is because E = 1 whenever q > 0. So, as mentioned above, the equation for total future service can be simplified to the value shown in column (B). Column (C) illustrates the full equation and verifies that we do, in fact, get the same result. Furthermore, average future service is the same as total future service because the denominator is 1 (again, because E = 1 whenever q > 0). Also note that nothing is included in the summation of future service for the age 55 row, because t = 0 at that age and the summation starts at t = 1.
An algebraically equivalent way to determine the total future expected working lifetime is based on the formula below. This formula is the basis for the format used to develop the expected future working lifetime in the sample life report.
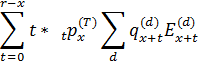
In other words, for each possible future decrement year, t, multiply the number of years of service achieved at decrement by the fraction of a participant expected to terminate with a benefit during the year.
| (A) | (B) | (C) | (D) | |||||
| Age | q(m) | E(m) | q(r) | E(r) | q(m)*E(m) + q(r)*E(r) | p(T) | t | (A) * (B) * (C) |
| 55 | 0.008 | 1 | 0 | 0 | 0 | 0 | ||
| 56 | 0.009 | 1 | 0 | 0 | 0.009 | 0.992000 | 1 | 0.008928 |
| 57 | 0.01 | 1 | 0 | 0 | 0.01 | 0.983072 | 2 | 0.019661 |
| 58 | 0.011 | 1 | 0 | 0 | 0.011 | 0.973241 | 3 | 0.032117 |
| 59 | 0.012 | 1 | 0 | 0 | 0.012 | 0.962536 | 4 | 0.046202 |
| 60 | 0.013 | 1 | 0.2 | 1 | 0.213 | 0.950985 | 5 | 1.012799 |
| 61 | 0.014 | 1 | 0.1 | 1 | 0.114 | 0.748425 | 6 | 0.511923 |
| 62 | 0 | 0 | 1 | 1 | 1 | 0.663105 | 7 | 4.641735 |
| Total | 6.273364 | |||||||
| Average | 6.273364 |
The result of this calculation is identical to the one shown above since it is algebraically equivalent. Column(C) is the number of years of expected future service achieved by a person decrementing each year. Multiplying (C) by the probability of decrementing gives us the expected future service.
Expected Service Lives (under GASB 68 or 75)
To calculate expected service lives for GASB 68 or 75, ProVal uses the numerator formulas for the total future service option (shown above) and sets E to 1 until the last decrement age at which a non-zero benefit is assumed to be payable and zero for all decrement ages thereafter. For actives in all modes and inactives in OPEB mode, the denominator is set to 1 if any non-zero benefit is assumed to be payable at any decrement age and zero otherwise. For inactives (including vested-value-as-actives) in pension modes, the denominator is set to 1.
Determining E (not applicable to expected service lives under GASB 68 or 75)
In the formulas above, E is 1 if a positive employer-provided benefit is projected to be payable upon decrement (E is 0 otherwise).
In ProVal, E is 1 for decrement d (retirement, termination, death or disability) at decrement age x+s if, for any benefit associated with decrement d, all of the following are true:
-
The member is eligible at age x+s (eligible at mid-year if middle of year decrement timing is assumed),
-
the probability of decrementing and receiving a benefit at age x+s is greater than 0,
-
in the pension modes only, the benefit amount at decrement age x+s is greater than 0, and
-
in OPEB mode only, the benefit amount at decrement age x+s is nonzero. When determining the benefit amount, ProVal separately looks at the Gross Benefit Definition and the Participant Contribution; they are not netted together. Therefore, participants valued in an “access-only” plan, where the Gross Benefit Definition is the same as the Participant Contribution, will have a zero EPBO and a non-zero expected future lifetime (unless the participant is at the 100% retirement age on the valuation date).
One could ask if further adjustments should be made for other circumstances where benefits are not payable, for example, if a preretirement death benefit (e.g., a REA benefit in the U.S. qualified mode) is payable for married members but not for single members. The response is that ProVal determines E by looking for any benefit payable for decrement d occurring at decrement age x+s. It does not attempt to make further refinements if additional factors applied after decrement do not sum to 1 (such as election probabilities or the fraction assumed married). Per Actuarial Compliance Guideline No. 1, “Approximations will generally be satisfactory, especially where the decrements for which benefits are or are not payable are very small. For example, a plan might provide only a disability benefit for terminations with less than 10 years of service, and provide benefits for all terminations except unmarried deaths after 10 years of service. It would normally be acceptable to treat E for all decrements as 0 in the first 10 years and 1 thereafter under these circumstances.”
Also, would it be appropriate to count some decrements as “half benefiting”, for those who were “benefiting” at $0 at the beginning of the iteration age but not “benefiting” at $0 at the end? That is, use an “average” of benefiting E’s as of the beginning and end of the iteration year in the numerator and in the denominator. While it would be reasonable, ProVal does not take this more detailed approach.
Asset & Funding Policy
Under the Future Amortizations button of the Accounting Methodology topic, there is an option that allows you to select the method used to compute the denominator for the average future service or life expectancy (whichever you have chosen as your amortization basis). Note that if you select “Average headcount during the year”, results under the amortization basis (either average future service or life expectancy) might not match the Output values for your Valuation or Core Projection, because the Valuation or Core Projection run had calculated the average using the “Headcount expected to receive benefits” option. For more information, see the discussion of this parameter in the What’s New article for ProVal Version 3.01.